Semi-Bluffing By Raising All In

26 Feb, 2011
The game is $50NL. Our opponent is a regular with a VPIP/PFR of 24/20 and a 3bet of 9%. So they seem to be a tough and aggressive player.
The hand plays out as follows...
BTN ($50)
Hero SB ($50)
BB ($50)
Preflop: Hero is SB with


BTN folds, Hero raises $1.50, BB raises $4.50, Hero calls $3
Flop: ($9)


 (2 players)
(2 players)Hero checks, BB bets $5, Hero raises $45.50
How can we work out if this semi-bluff all-in raise is profitable?
First, lets do some PokerStove work.
When we check-raise all in, the hands she calls with are TT-AA, ATo, T9s, T7s.
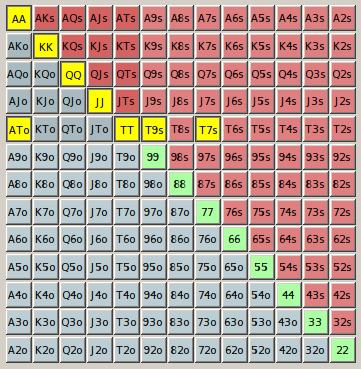
Tx hands.PokerStove shows that when called by the above range we have 20.6% equity. And therefore we must lose 79.4%.
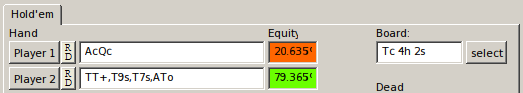
PokerStove also shows that the hands she calls with when we check-raise all in represent 2.5% of starting hands (it actually represents 3.8% at first, but we hold the Ac and Qc, and the Tc is on the flop).
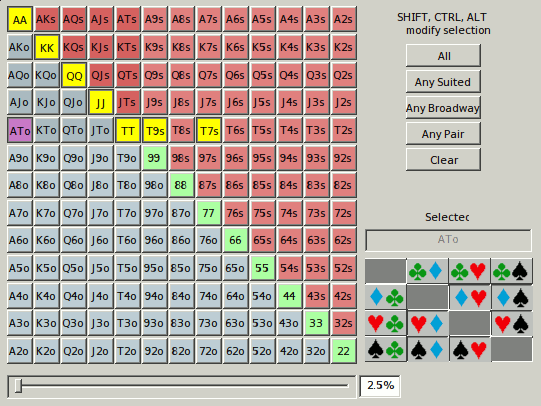
Ac, Qc, Tc from the initial range we gave her (because they're blockers), which gives us 2.5%.Therefore she calls us with 2.5% of hands and she 3bets with about 9% of hands, so she calls our shove 2.5/9 = 28% of the time (in fact, she is probably 3betting the most when the SB opens into her, but for argument's sake we will use 9%).
What is the EV of getting the money in?
When we get it all in and win, we win the $9 currently in the pot plus the $45.50 that we raise. When we lose, we just lose the $45.50 that we raised to. So we can calculate our all in EV as follows:
EV all in = [(9+45.5) * 0.206)] - (45.5 * 0.794)
= 11.2 - 36.1
= -24.9
So our EV every time we actually get the money in is -$24.9.
That's obviously not good. How do we make money?
Well, here we need to do some more analysis. When she folds we win $14, because there was $9 in the pot and she bet $5, but now folded. This is really important.
We already calculated that because of the range of hands she calls us with compared to range she 3bets with preflop, she will call us 28% of the time and fold 72%.
Therefore we can calculate the EV of check-shoving this flop quite easily by creating a formula. All we need to is compare the EV of when she folds with the EV of when she calls.
EV of check-shoving.
Since we know she folds 72% of the time and we win $14 when she does, we can use (0.72 * 14) to represent the part of the equation where she folds. And since we know she calls 28% of the time and we lose $24.9 on average when she does, we can use -(0.28 * 24.9) to represent the part of the equation where she calls.
Thus we can combine the two parts of the equation and get:
EV of check-shoving = (0.72 * 14) - (0.28 * 24.9)
= 10 - 7
= +3
So you win roughly $3/hand, or about 6bb/hand by check-shoving this flop.
Whilst that might not sound a lot, in terms of winrate, adding 6bb/hand to your winrate in a couple of spots makes a dramatic difference, see breaking down your winrate for the reason why. However, you can probably just accept right now that some people don't even win at 6bb/100, and here you are winning all those 6bb in one hand!
Of course you can adjust the figures in this post to be able to see how various factors affect the profitability of shoving:
- What if we have
AJ? - What if we have a backdoor flush draw?
- What if she 3bets more/less?
Using the process and formula above will allow you to create any situation you like, providing you go to PokerStove to work out your equity and the amount of times your opponent can call.
Does this mean you should always call a 3bet with AQ and check raise all in on these type of boards? No. If you are playing someone who is tight and only re-raising about 6% in this spot, you will find it to be quite -EV to check raise all in here as you will frequently get owned by an overpair.
The idea of this article is to get you thinking about how you can apply maths away from the table to other similar spots.
Jack.
